I.8
10/2/2021
Warm, soft reddish flesh, pierced by a prismatically blue icicle.
So in total, finishing the book would take at least 40*2*10=800
days. That's OVER 2 FUCKING YEARS.

Is that it? Is that algebraic geometry?
I wrote those words back in November of last year, and now here we are: the end
of chapter 1. Whew. Kinda surreal. 98 posts of algebraic geometry, and we made
it.
this blog, Hartshorned, has been a very intense project. it takes hours to make
each post, on top of the time it takes to do the math, and i post multiple times
per week. And thus, it's had some ups and downs, lol. Forgive me for the downs.
tbh I actually almost deleted the site numerous times along the way (*ahem* the
infamous redactions, and other stuff), but here we are, ALIVE. thanks to those
who gave followed along, gave feedback, etc.–I would have probably given up
otherwise.
Now it's time to figure out if we should continue to the next chapter in
in my life in the book. WILL HARTSHORNED
CONTINUE? and if so, when? (the rest of this site will def be updated regularly
tho, dw). Let's start with Hartshorne's question:
"What is algebraic geometry?" - Ummm, IT'S ABOUT TIME, QUEEN. Maybe
you coulda explained this a bit earlier??? Well, anyway let's find out:
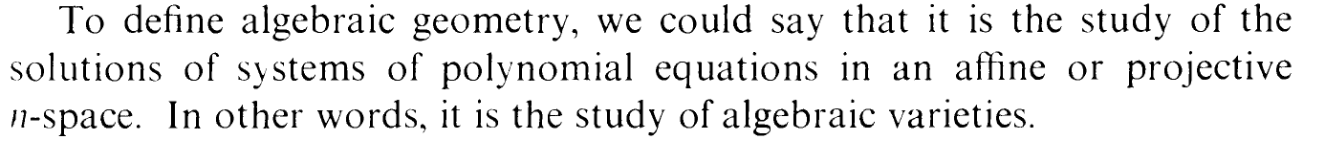
BOOOOOOOOORING. THAT'S IT? TIME. WASTED.
Okay, I'm just kidding. It gets a little more interesting when he elaborates:


So you can describe an entire class of varieties, by using another variety!? Okay:
So you can make a variety, WHERE EVERY POINT REPRESENTS ITS OWN
VARIETY?? THAT IS SO FUCKING META. SO YOU CAN TAKE ALL THE
GEOMETRIC FIGURES IN A BIRATIONAL EQUIVALENCE CLASS, AND
YOU CAN REPRESENT IT WITH ANOTHER GEOMETRIC FIGURE. IT'S
LIKE, META-GEOMETRY. HOLY FUUUUUCK. Too bad I wasn't planning
on going all the way up to Chapter IV LOL. but now i kinda want to
(also that's where elliptic curves comes up in detail, which is a thing)

In my very second post on this blog (my early posts are cringe. do not read), I did
some calculations:
It's been a year, and I've finished only chapter 1. SAY IT WITH ME: "The
purpose of this blog is not to get better, but to get worse" lol. why does that feel
so good to say.
Now, chapter 3 is kinda like my main goal for this book, cause it involves
cohomology, i.e. arrows n stuff: indeed, commutative diagrams (like this ) have
always been very alluring to me, even if I don't know why they're useful. if I'm
moving forward in this book, it's probably good to make some observations on the
past year:
- I think I did too many exercises per section. I did almost every exercise in sections 1-5, and more than half for sections 6 & 7. If I continue onto chapter 2, I think "more than half" is probably a better amount
- I also spent too much time per exercise? Like often I'd spend 3 days on an exercise only to look at the solution. I should probably put a limit on that. Tho it might take away from the euphoria of reaching a breakthrough and figuring something out after 3 days. SACRIFICES MUST BE MADE.
- Hartshorned began on 11/22/2020. So if I am doing a chapter 2, maybe I should start on 11/22/21. That way I can use my chapter 1 progress as a benchmark
- Honestly, even if it took 1 year per chapter, I'd be done with chapter 3 in "only" two years. That's better than having nothing in two years. I won't even be 30 by then, kek. sometimes I feel like I'm getting old, but I have to remember I'm still actually kinda young
- hopefully other people that work on this book/do mathematics can look at my big list and see the progression of exercises by an exceptionally unproductive person. if i can do it, you can
- one well known caveat of learning is that "if you don't use it, you lose it." However, the fact that I've recorded every exercise makes me less worried about that. I've been able to look at very old exercises and remember how to solve them because I wrote it down in my own words. If I forget something, I can follow my own instructions to reproduce it. Look at this or this (man, I remember how long they took to do. sitting in my bed, scribbling in my notebook and typing it out). Even though they're gigantic messes, they're more understandable to me than the slick, abstract, "better" solutions that more advanced people put out.
- I should state that the highest level of math I took was Calc 3. Everything beyond that has been pure self-study. My topology knowledge has been pretty sufficient for this book, but my abstract algebra knowledge... oh boy. I wonder if I should brush up on that
- chapter 2 has 9 sections, but only the first 5 are fundamental according to our queen, so that should maybe shorten it?
- If you have any questions or comments about how i've maintained this blog, let me know. I'll be glad to respond. my contact info is on the about page

Here's 3.13. Lol, you see? it's fun to just be able to casually link an old post at the
snap of a finger. been there, done that, bitch. also that last part is funny because
this book was apparently published in 1977. Fermat's last theorem was solved in
1994. Things take time. btw, the reason our Queen mentions non algebraically
closed fields is to motivate SCHEMES, which is what chapter 2 is about.
So Hartshorned will probably go on hiatus at least till 11/22, and we'll see then if
I (and you, reader!) want to embark on chapter 2.
REGARDLESS, i will be regularly updating the rest of the site. If Hartshorned is
on hiatus, i promise i'll put that same amount of effort into whatever else takes its
place on here (tho mayb after a smol break lol). I hope you'll follow along
for w/e i do next, thru the ups and downs. Time for some wildcards.
Reader: Thanks for following along. I know this was a very weird thing to follow
along to. Did you enjoy the ride?
And thus, chapter 1 is DONE.