HEEEEEEEEEEEE'S BACK! THE QUEEN. The Man! The Mathman. Robin Hartsho–American Robin. WHEW. THE BIRD ENJOYER. The EASY LAY (proof not provided). Sell your tickets to Spoder and knock on #6f1623. Yus, yus. KNOCK KNOCK! FREE ENTRY. FREEEE DICKSUCKING. BOYTOY FOR SALE. HEEEEEEEEEEEE'S A GROWER. I'll take your money as a donation, thank you very much >He does it for free. What? FREE? FREE MATH!? SUE ME FOR MAKING A SOLUTIONS GUIDE. Listen: I can't solve your life. I can't solve the hole in your heart. You're stuck with it, ma'am. Ma'am, I'M SORRY. I CAN'T. I cannot fill that divot. I can pour warm coffee in it, but it'll be evaporated by your lungs soon leaving that lasting want. Now, now, ma'am, don't get flustered. Hold it, ma'am, stop approaching me, those little knockers of yours are going to smother m–MMFMFMFFEMFMFEFM ACCHCUHEHUCEHUECHCEUCHUEHUC I'M CHOKING, ARRRRRGGGGHGHHH! THE DASTARDLY PERILS OF THE BARELY MOUNDED BARE CHEST. HEAVENS! I MUST ESCAPE TO MY APARTMENTS BEFORE IT IS TOO LATE! AH, THESE WRETCHED DEVICES. MY BOWELS ARE BEING REORGANIZED. S'BLOOD! O'ER THE LAND IT PREYS AND LEAVES US WANT OF GAIETY. THE BETRAYER OF SOULS. CURSE THESE BARREN PLAINS, WANT OF EXULTATION. O MA'AM, HAVE MERCY, AND LISTEN: Listen: I cannot solve that problem. That man behind the man: The elusive source of all your freefloating anxiety. NAY, we shan't unravel your deepest issue on this journey. You're not getting any better from reading on. Sorry, reader. TOO BAD, READER. Your misery shall stay. SUCKS FOR YOU, READER. I can't solve THAT problem; that's up to you. But I can solve THIS problem; this exercise, yes yes. Let's take a look:

–WAIT, HOLD ON. We have some setup to do. First, let's give a SHOUT OUT to Linky, koshka, and The King in Yellow for inspiring this color scheme. ROUND OF APPLAUSE! *Clap clap clap clap clap clap clap*. And most of all, let's congratulate ME, holeinmyheart, for diving into this project again, that I am already starting to regret because NOW I REMEMBER HOW DIFFICULT IT IS TO DO THESE POSTS *Clap clap clap clap clap clap clap clap clap clap clap clap clap clap clap clap clap*

But as they say: The HART MUST BE SHORNED. and here we are: A self-motivating prophecy. AND, since I've wisened up (but not smartened up, which shall serve to be a problem), I should probably introduce the chapter and section before hopping straight into the exercise:



DUN DUN DUN (A cursory look at the history of alggeo will tell you this is a big deal). But before we get to THAT, we have to deal wiiiiiiith:



Ugh.... UGH. Well, what is a sheaf? Well, first we have to define the notion of a presheaf, which is given here:



Just kidding lol (no but srsly in hindsight if you think about it it actually makes sense). Here's the non-meme definition:



Okay, so """intuitively""" a presheaf keeps track of "local data" on a topological space... or something. and a SHEAF is just that with the XTRA condition that you can "GLUE" elements together in a consistent, UNIQUE manner:



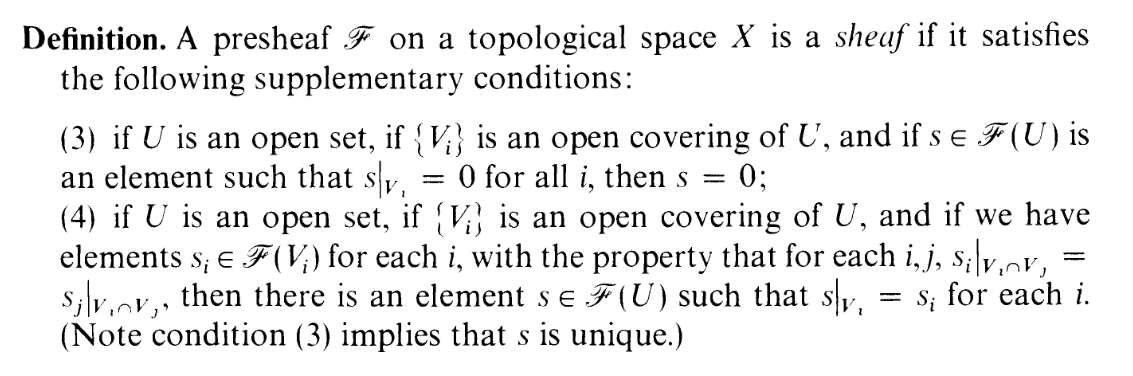
Annnnnd what we've learned is once you define OBJECTS in math, it's obligatory that they come with MORPHISMS:



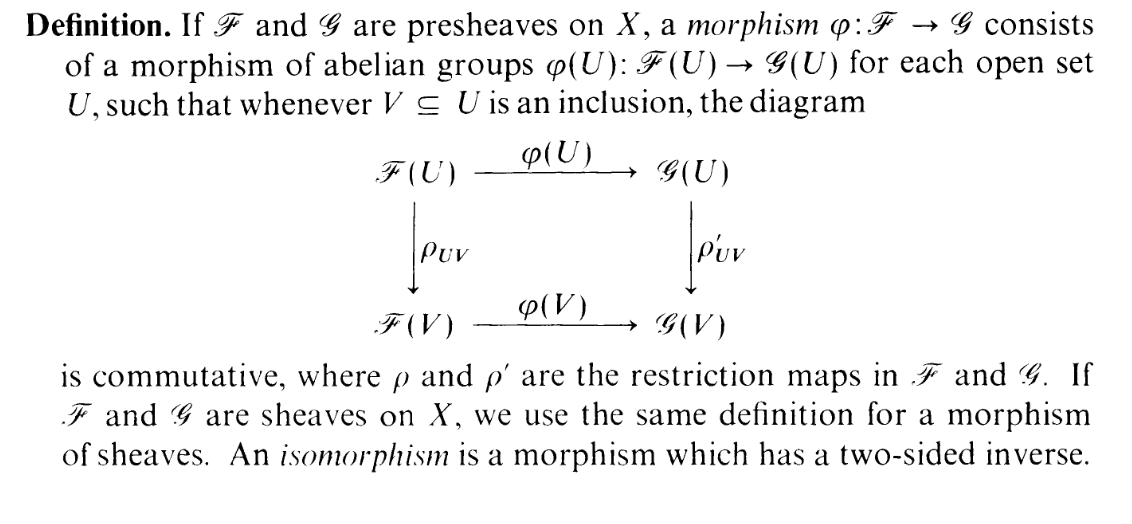
Finally, in the spirit of... "locality" i guess.... you can zoom into individual points and obtain "stalks":



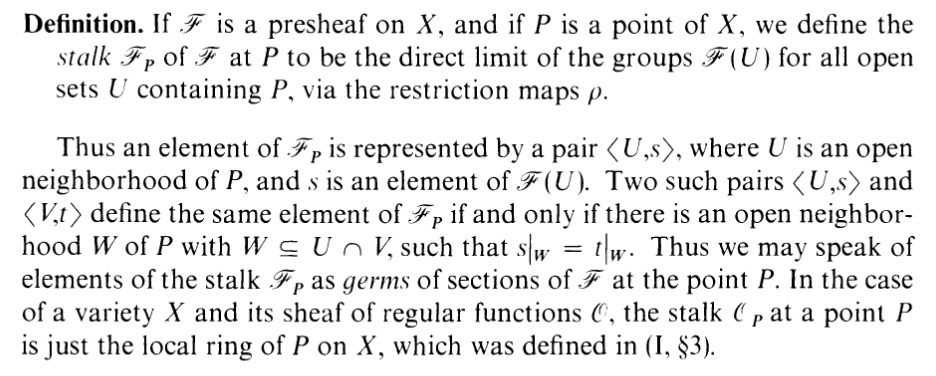
So those are our basic definitions. ONTO THE EXERCISE:



Okay, so this requires... more definitions. here's the definition of the constant sheaf:



And we also need the definition of the "Sheaf associated to a presheaf" (LMFAO sorry folks, the early exercises in each section tend to devolve into definition spam . But this is the last one for this one):



I'm going to call the above process "sheafification" cause that sounds nicer than "sheaf associated to presheaf" lol.

OKAY.... FUCKING FINALLY. WE CAN NOW START THE FUCKING EXERCISE. FUCK, THAT WAS TOO MANY DEFINITIONS.

So I want to show that the SHEAFIFICATION of their "constant presheaf" associated to X (U A,∀U≠0) is isomorphic to the constant SHEAF associated to X. (BTW this is retarded so skip this parenthetical but if I didn't use "sheafify" I would have had to say "the sheaf associated to the constant presheaf associated to X" lolololol fucking word salad)

Fuck man. I haven't even started the exercise and i'm exhausted from typing/formatting all that. BUT THE HART MUST SHORNED. TIME FOR SHORNING.

Denoting the constant presheaf as C, note that clearly given P ∈ X the stalk of P is "clearly" C_P = A

Now, by definition, the sheafification C⁺ of C is given by

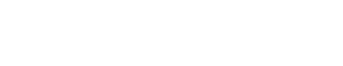(1)

where the s satisfy the sheafification conditions from above.

ALRIGHT. First thing's first. Note:

⋃ P∈UCP	= ⋃ P∈UA
	= A

BTW: That last equality works because implicit in the definition is that we're applying the equivalence relation across C_P's. What I'm trying to say is that we can identify elements in one C_P = A with the ones in another C_P = A, so we DON'T just get a disjoint union of As. We get one copy of A, not one for each P. YOU KNOW WHAT I MEAN? LOL. The reason I know this to be the case is because this is the only way the exercise actually works out.

HENCE, I can write (1) as:

C+(U)

= {s : U → A}

This is almost the constant sheaf definition. The only thing missing is the stipulation of continuity. Soooo, let's take a look at those sheafification conditions:

1. s(P) ∈ C_P = A... which is redundant
2. ∀P ∈ U,∃ a neighborhood V ⊂ U of P and t ∈ C(V ) = A such that
   ∀Q ∈ V : t_Q = t = s(Q). So basically s(V ) = t

So (2) is supposed to help us out with continuity... SOMEHOW. This is where I got stuck for 1-2 hours.

Ahhh, getting stuck on the first exercise. As expected from the master, holeinmyheart.

So basically here's the issue. With A having the discrete topology, continuity means we need to show that for any t ∈ A, S = s^-1(t) is open. Now (2) is ENTICING in the sense that we DO have open sets that map to t... but it's not necessarily the case that the entire preimage of t is open. That "V " is just a subset of S. How do we show that S itself is open? That's where I got le stuck. But... I kinda answered my own question here, didn't I? I have an open subset V of a set S. If I can cover S with such open subsets, I'm good. Yep yep!

So we have our map s : U → A. And we're looking at S = s^-1(t) ⊂ U. By condition (2), given P ∈ S, there is a neighborhood V _P of P contained in U such that s(V _P) = t. Hence V _P ⊂ S so ⋃ _P∈SV _P = S is an open cover of S. Hence S is open and s is continuous. DONE!

Alright, that's our first exercise, reader. Seeya in the next one.