II.1.2
12/18/2021
So I just saw the new Spiderman movie. The nonspoiler version is: It was
FUCKING AWESOME. I loved how they brought back the old cast in a way that
wasn't too on the nose. And yes, this is an absolute must-see in theaters. The audience's
reactions for some of the reveals were honestly something that I won't forget any
time soon. So yeah, highly recommended. I'm going to get into spoilers now, so if you
haven't watched it definitely do so before reading this post.

Alright, spoiler time. Okay: I'm usually not one for nostalgia bait, but I'll admit
when Andrew Garfield came on screen and the audience fucking screamed, I was
grinning like an idiot. Collectively realizing we were getting a full-on multiverse
movie was just a magical moment. And the fucking Tobey Maguire entrance? I
kid you not: People were fucking jumping out of their seats when he appeared. I
can't believe they pulled it off. What a fucking time. It was just a constant
hype train from there. Seeing all the old villains gang up was also just
pure bliss. Willem Dafoe was just excellent. Holy shit, what a ride. I
wish I could go back and see it for the first time again. One of the best
theatergoing experiences of my life, by far. Though what was up with that 10
minute rant in the middle by Tobey about how "Harvey Weinstein was
innocent"? That was seriously inappropriate. How did that even get past the
Hollywood censors? It's categorical misinformation. Everyone knows
Harvey Weinstein is guilty. He was convicted by a jury in a fair trial, after
all. But Tobey just kept going on making these false claims about how "He
was set up" and "There was no actual evidence" and how "He literally
did nothing wrong." It didn't even have anything to do with the plot.
I remember Tom and Andrew tried to calm him down but Tobey just
replied "My twink ass has been in Hollywood longer than yours," and
the audience clapped for some reason. That line makes no sense in the
context of the movie's universe. It was clearly just Tobey adlibbing or
something. I know he got a big check, but were they that desperate to get
him on screen? Seriously, I just don't think it's befitting of Peter Parker to tout conspiracy theories about how "Big Bad Harv" was "unfairly
persecuted by the media" for "consensually tearing up goypussy." Putting
these sorts of lies on the big screen is at best irresponsible and at worst
dangerous. To his credit, Tom tried to play along and said "I see where you're
coming from. That J. Jonah Jameson guy really gets on my nerves," but
Tobey just smirked and said "Kid, when you grow up, you'll realize he's
based" then started ranting about some journalist that wouldn't call him
"Tugboat Maguire." At that point Zendaya finally had enough and called
Tobey's comments "disgusting," but Tobey just replied "Hey lady, how bout
you get me some milk and cookies?" and the audience clapped again.
Extremely misogynistic. Did Sam Raimi write this scene or something?
A
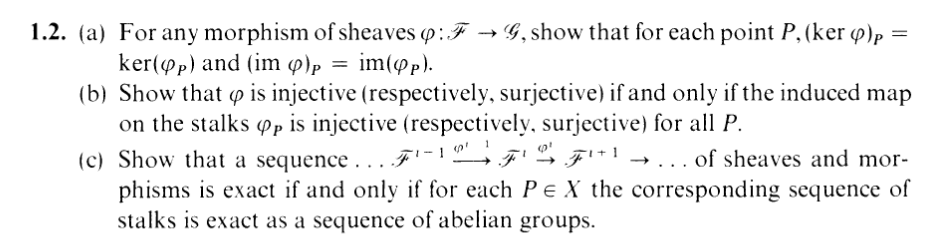
Today's exercise is very cursed. Well, okay, Part B and C are, but part A is alright. What
we're doing, in a broad sense here in 1.2, is relating sheaves to stalks. oh, and here's your
sheaf kernel bro:

So the sheaf kernel is just the presheaf kernel, which has a "natural" definition.
Cool. That makes part A not too bad. What I'm gonna do is take the map
| δ : (ker ϕ)P | → ker(ϕP) | ||
| [U,s] |
 < U,s > < U,s > |
and show that it's an isomorphism.
(Note my use of the different brackets to differentiate. I will do this in the later
parts).
0.1 Cute and valid?
Well, is this even a valid map? First of all: Is "< U,s >" even an element of
ker(ϕP)? Well, since [U,s] ∈ (ker ϕ)P, I know that s ∈ ker ϕ(U) ⊂
 (U),
so < U,s > is actually a valid member of
(U),
so < U,s > is actually a valid member of
 P. YOU ARE VALID, < U,s >.
And furthermore it is in fact trivially a member of the subset ker(ϕP) ⊂
P. YOU ARE VALID, < U,s >.
And furthermore it is in fact trivially a member of the subset ker(ϕP) ⊂
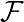 P.
P.
Okay, there's another thing we have to check since we're working with equivalence
classes: Well-definededness Let's say that [U,s] = [V,t] Then we have a
neighborhood W of P such that s|W = t|W on ker ϕ(W) ⊂
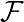 (W), hence
< U,s >=< V,t > in
(W), hence
< U,s >=< V,t > in
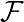 P, which ker(ϕP) is a subset of.
P, which ker(ϕP) is a subset of.
0.2 Surjectivity
Suppose < U,s >∈ ker(ϕP) ⊂
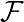 P. So s ∈
P. So s ∈
 (U), and
(U), and
| 0 | = ϕP(< U,s >) | ||
| =< U,ϕ(U)(s) > |
So BASICALLY, I can assume that ϕ(U)(s) is 0 when restricted to some
open set V . I.e. denoting the restriction maps as ρ, I know
| 0 | = ρU,V (ϕ(U)(s)) | ||
| = ϕ(V )(ρU,V (s)) |
by commutative property of morphisms. HENCE, ρU,V (s) ∈ ker ϕ(V ), and
thus [V,ρU,V (s)] ∈ (ker ϕ)P, so..
| [V,ρU,V (s)] |
 < V,ρU,V (s) > < V,ρU,V (s) > | ||
| =< U,s > |
0.3 Injectivity
ok this one is easier lol
| δ([U,s]) | = δ([V,t]) | ||
| ⇔ < U,s > | =< V,t > | ||
| ⇔∃W : s|W | = t|W in
 (W) (W) | ||
Since ker ϕ inherits the restriction maps of
 , the last equality also holds in
ker ϕ(W). Hence [U,s] = [V,t] in (ker ϕ)P. DONE.
, the last equality also holds in
ker ϕ(W). Hence [U,s] = [V,t] in (ker ϕ)P. DONE.
"Umm, what about the im part"... Ummm, I'm too lazy. I bet it's analogous
anyway lolololol.
B
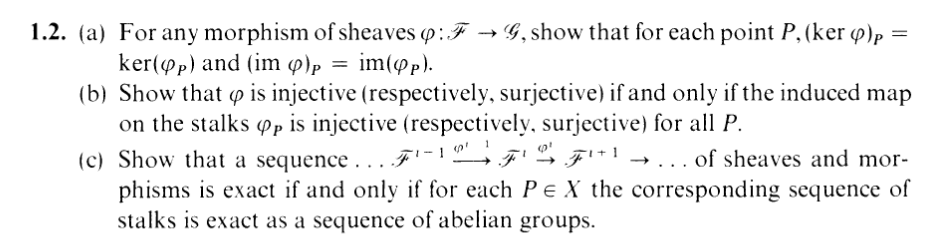
I almost vomited when I saw this part. There's already a proof in the book that
proves almost the same thing but not quite. In fact, the closer you look, the more
you realize this is QUITE a different beast. Y'see, before you get ahead of yourself
and start working with the sectional maps, you have to ask: How are injectivity
and surjectivity even defined for sheaf morphisms? Here's injectivity:
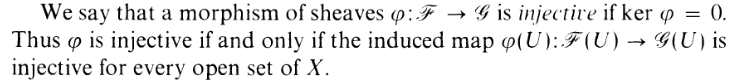
Okay, common sense! Works the way you expect. What about surjectivity?
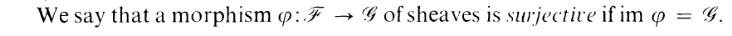
Now, I've heard certain dweebs
complain about things like how the "quotient rule" is unintuitive and
such. Hahahahahaha, cute. Let's just say they haven't seen true horror.

AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA
WHYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYY
So yea basically at this point, I just said "fuck it, I'm skipping this" and went on
to the next part... Turns out... That was (unintentionally) a 999 IQ play. Watch
this:
C
BTW: In case you're wondering, I didn't actually watch the new Spoderman
movie lol. Spider-Man 2 (2004) is probably one of my favorite movies though.
(And... fuck. can I just say Tobey Maguire is cute. "Ummm, you know he's a
complete asshole in real life?" THAT. JUST. MAKES. IT. BETTER??? THE
CONTRAST BETWEEN HIS BOYISH FACE/VOICE AND HIS FUCJING
DOUCHEBAG PERSONALITY YES YES YES YES PLEASE). But yeah that
aside I just can't get excited over this sort of stuff enough to buy tickets or even
pirate it and watch it. I legitimately mean it when I say there is something
admirable in the way people can get excited over the newest superhero
movie or AAA game or anime or whatever, because over the past few
years I've basically lost so much interest in media in general. I just no
longer feel the joy of consooming. I don't just mean pop media either. A
while back I tried playing a new VN by one of my favorite writers and I
just dropped it in the middle even though I love his writing. I've just
lost interest in everything. And it's not like I "dislike" myself for being
like this (I have other reasons to dislike myself, lmao)–I kind of know why I'm like this now and why it has to be that
way–but seeing everyone excited over things makes me feel kinda lonely.
"Ummm is this your 999 IQ play?" No, my 999 IQ play is this:
MUAHAHAHAHAHA, DON'T YOU SEE READER? IF I DO PART C, IT
AUTOMATICALLY PROVES PART B............ WHICH MEANS
HARTSHORNE WAS TRYING TO MAKE ME DO EXTRA
WORK. WHAT THE FUCK.
Okie dokie, Let me first expand that and give you a whole bunch of new
definitions:

Okay, so like injectivity, the kernel works the way you expect. However, the
image–since it relates to surjectivity–doesn't. Right: Note that the SHEAF
IMAGE is NOT U
 im(ϕ(U)). That is the PRESHEAF IMAGE. The
sheaf image is the SHEAFIFICATION OF THE PRESHEAF IMAGE.
im(ϕ(U)). That is the PRESHEAF IMAGE. The
sheaf image is the SHEAFIFICATION OF THE PRESHEAF IMAGE.
You know what that means, motherfuckers? YOU WANNA
SEE TRUE HORROR, MOTHERFUCKERS? GUESS
WHAT. IN GENERAL,
| (imϕ)(U) | ≠im(ϕ(U)) | (1) |
AAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAAA
FUCKING WHYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYYY
Yeah, so if I denote the presheaf image as imp, then the right hand side of the
above inequality is (impϕ)(U). It's slightly better than a straight up
inequality though, as the RHS is a (possibly proper) subset of the LHS.
Okay, let's do this. We're comparing sequences
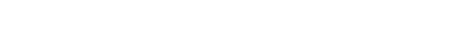 | (2) |
 | (3) |
(P ∈ X) Let's start with the only-if:
0.4

Let's suppose (2) is exact, so
| ker ϕi | = imϕi-1 | (4) |
We want to show that (3) is exact for arbitrary P ∈ X, i.e. we want to prove
the equality
| ker(ϕPi) |
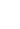 im(ϕ
Pi-1) im(ϕ
Pi-1) |
BTW, note the parentheses. Alright. So let's take an element < U,f >∈ ker(ϕPi), so that
| f | ∈ (ker ϕi)(U) | |||||
 f f | ∈ (imϕi-1)(U) | (4) | ||||
which does NOT necessarilly mean f ∈ im(ϕi-1(U)) thanks to the
inequality of doom (1). But imϕi-1 is the sheafification of impϕi-1, whose
sections are indeed im(ϕi-1(V )). So by the sheafification construction, I can
claim that ∃ a neighborhood V ⊂ U of P, and a t ∈ im(ϕi-1(V )), such that
t = f|V . Hence < U,f >=< V,t >∈ im(ϕPi-1).
Hence ker(ϕPi) ⊂ im(ϕPi-1)
What a fucking mess. The other inclusion is a bit better. Given
< U,f >∈ im(ϕPi-1), again, I can get a section t such that < V,t >=< U,f >
and
| t | ∈ im(ϕi-1(V )) | |||||
| ⊂ (imϕi-1)(V ) | ||||||
| = (ker ϕi)(V ) | (4) | |||||
so < V,t >∈ ker ϕPi. Whew.
0.5 ⇐=
ALRIGHT. LAST STRETCH. So now I'm supposing that (3) is exact ∀P ∈ X
and I want to show (2) is. I.e. I know
| ker(ϕPi) | = im(ϕ Pi-1) |
for all P ∈ X, and I want to show
| ker ϕi |
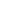 imϕi-1 imϕi-1 | (5) |
So given f ∈ ker ϕi(U), I know that
| < U,f > | ∈ ker(ϕPi) | ||
| = im(ϕPi-1) |
which means ∃ < V P,gP >∈
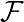 Pi-1 (gP here is the section, not the stalk
element) such that
Pi-1 (gP here is the section, not the stalk
element) such that
| ϕPi-1(< V P,gP >) | =< U,f > | ||
 < V P,ϕPi-1(V
P)(gP) > < V P,ϕPi-1(V
P)(gP) > | =< U,f > | ||
Now I'm gonna denote hP = ϕPi-1(V P)(gP). Refining if necessary, we can
assume that f|V P = hP. And since
| hP | ∈ im(ϕi-1(V P)) | ||
| ⊂ (imϕi-1)(V P) |
we can glue the hP's together to form an element h ∈ (imϕi-1)(U). But by
uniqueness, we must have h = f! Hence, ker ϕi(U) ⊂ imϕi-1(U)
LMFAO. As I type this I realize I haven't done the reverse inclusion yet. I felt so exhausted
that I felt I was done already. FUUUUUUUUUUUUUUUUUUUCK I'M SO FUCKING
TIRED FUCK FUCK FUCK FUCK FUCCKKKKKKKKKKKKKKKKKKKKKK.
OK, QUICKLY: Suppose f ∈ (imϕi-1)(U). Then for each P ∈ U,∃V P,gP
such that f|V P = gP, and
| gP | ∈ im(ϕi-1(U)) | ||
 < V P,gP > < V P,gP > | ∈ im(ϕPi-1) | ||
| = ker(ϕPi-1) |
AND THEN REFINE EM, GLUE EM, AND YOU'RE DONE! SEEYA IN THE NEXT EXERCISE READER.