I.2.9a - Errata
2/11/2021
*AHEM* Remember that C is irreducible
Hole in my heart? More like hole in my proof. Yes, reader, just remember.. You remember, right? C is
irreducible.... C is irreducible.... You're being hypnotized.... Yess..... Now....... lick my dick..........
Talk about an asspull. Yes, I pulled that one out of the hole in my ass, persay. For whatever reason, since
Z(β(T′)) ∩ U was irreducible, I assumed that Z(β(T′)) was irreducible. NOT necessarily true.
Z(β(T′)) is merely a closed set. There's not much reason to assume it's irreducible right off the bat.
Well, what do then? It's thanks to that asspull that I was able to say
 | (1) |
and perform a series of steps that got me to
 | (2) |
HOLD ON. The first equation is an equality, but second equation is an inclusion. There might be some wiggle room here. Check it out:
| Y | = clPn(Z(β(T′)) ∩ U) | |||||
| ⊂ clPn(Z(β(T′))) | ||||||
| = Z(β(T′)) | (closure of closed set) |
| I(Y ) | ⊃ I(Z(β(T′))) | (I reverses inclusion) | ||||
=
 | (Nullstellensatz) | |||||
| ⊃ Sβ(T′) |
And there's (2) again.
Phew, my proof just barely hangs together. We are SAFE!
 One more thing:
One more thing:I asked you to take for granted that
 | (3) |
claiming that "it's in my notes" (lol), then proceeded to conclude.
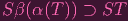 | (4) |
Let me justify that:
Grab some element f from T ⊂ Sh, like let's say (working in P3)...
| f(w,x,y,z) | = wxy + w2x + w3 + xyz | |||||
 α(f) α(f) | = xy + x + 1 + xyz | definition of α | ||||
 β(α(f)) β(α(f)) | = w3(

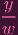 + +
 + 1 + + 1 +

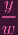
 ) ) | definition of β | ||||
| = wxy + w2x + w3 + xyz | ||||||
| = f |
| f | = wxy + w2x + w3 + wyz | ||
 α(f) α(f) | = xy + x + 1 + yz | ||
 β(α(f)) β(α(f)) | = xy + wx + w2 + xz | ||
| = f∕w |
Okay, so we don't exactly recover f the way we want, but if β(α(T)) were an ideal, then we could say that
| wrβ(α(f)) | = wr
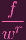 | ||
| = f |
