I.6.6ab
9/7/2021
I can hear it. The knocks. It's him. I knew I couldn't shake him
off. He always comes back. I'll have to escape out the window, sliding down the
rope like I've practiced over and over. Oh God, the knocks, the knocks.
The ear-curdling speech. By the time I've escaped, he'll have probably
found his way in. He. Always. Finds. A. Way. In. That's what people
don't understand about holeinmyheart. Ignorant shitters say "Just leeeave
him. Just ignooore him." But it's not that easy. Not with him. You can't
just shake him off. He'll fucking follow you, and he'll fucking ruin you.
God, he's probably going to read this. He's probably reading it right
now. Hey, holeinmyheart, if you're reading this: Fuck you. Fuck you. Fuck
you. Fuck you. Fuck you. You are everything that is wrong with this
world. You are the worst thing that's happened to me. You are a selfish,
narcissistic, worthless pile of shit. I hope you die, holeinmyheart. I genuinely
hope you die. No one likes you. Everyone knows how manipulative you
are. You're fully aware of how much you emotionally break people down,
and you even enjoy it. You're sick. You're an amoral freak. Fuck you.
Fuck you. Fuck you. Fuck you. Everyday I pray for your death. How the
fuck could you hurt people and just walk away smiling? How the fuck
could you make so many people suffer? You can't just Up-Throw someone
at 9% and Rest them off the stage. It's just not fair.
Only A and B today, folks. C shall be done another day, maybe. Also speaking of
A and B and B and A, AND NOW YOU SEE ANOTHER ME I'VE BEEN
RELOADED YEA-AH I'M FIRED UP DON'T SHUT ME DOWN. I'M LIKE A
DREAM WITHIN A DREAM THAT'S BEEN DECODED. I'M FIRED UP I'M
HOT DON'T SHUT ME DOWNNNNN. Omg, guys. It's ABBA: Reloaded.
Catchy AF. ABBAsed ABBA.
Now, reader. Will you leave me standing in the hall, or let me enter? ...Oh, the
hall.... FUCJ. Reader...? Knock knock. KNOCK KNOCK. Oi, oy, lemme in ye
little punk. Come onn. Come on y'little... Knock knock... Ah! Don't ABBAndon
me! Don't ABBAnish me! Let me hear your voice, in any form! ABBAlk at my
doorstep ABBArgain, and in this ABBArren hall I'd hold siege–ABBAttalion of
one! ABBArk at me like an grunting, untuned ABBAssoon, and I'll hark it were a
whistling ABBAmboo. The ABBArbs of love have ripped your voice ABBAre–a
beach flooded into ABBAy. I used to ABBAwl at your ABBArbaric ABBArrages,
but now it's like ABBAnter to me! Ahhhh, your slurry ABBArley, your
hoarse ABBAsil, mixed into ABBAtter and ABBAked with lye into aural
ABBAlm. Delicious, like ABBAnana split for my ears. Lemme hear it again!
......... Reader? Reeeeeeeeaaaaaaader. Knock knock. I know you're in there. I see
the lights on. Knocky knockyyyyy~. Hmmm, reader must be shy. Well, as I always
say, if they don't respond to knocky knocky, then you gotta picky picky. My back
pocket is always stashed for picky picky. Here we are–Snake Rake, tension wrench.
Kneel, penetrate, slide. Slide in, slide out. In, out... In... out... in... out. Scrubby,
scrubby, scrub the hole. torquey torquey, turn the plug–oh there's a spin—! ....
Eh? ...It's.. stuck. Oi, oi, reader, don't tell me you have a spool! Let's see here.
Pin 1, rattle. Pin 2, rattle. Pin 3.... is a cockblock. Oh, reader! A 3 to separate us
2! A pin twixt kin! How could you! Okie dokie, let's lift, lift, put your
arms in the air, counter-rotate.... WEEEEEEEEE THERE'S MY SPIN.
*Barges in* HELLO, MY SWEE–Oh, they escaped out the window.
Well, well, the picker becomes the scavenger, eh? Hmm, let's see... A yellowing,
toothmarked spooled apple core, standing up on a small wood round table. A
recent dine, eh? Yellowing, but not brown–ripped off like raw meat, wounds
wound fresh from a ravenous rampage. Muahaha, reader always used to cut their
apples with a knife in my presence. Yes, one eats differently in private. Eating
alone is a specially intimate experience–selfcestual–and I'm here for the
postmortem. What mess took place at this table for two? Sticky liquid
painting their face, skin stuck between their teeth, unashamedly picked out
by their bare fingers? Lick, lick. Mmuuuahhh! There it is, my indirect
kiss. Oh–oh, what's this? A fallen sock. Sniff, sniff. Ahhhhhhhh, what a
fragrance! I'll pocket this one–I am the sock gnome for this apartment
room, nay, the sock puff. Yes, I've huffed and puffed my way in, and I'll
huff and puff my way through. Skip, skip. What a lovely playhouse! I
could frolick here all the way till their return. Hm, what's this? A diary?
A

SO, we're identifying P1 with A1 ∪{∞}: given a point (X,Y ) ∈ P1, let
X∕Y ∈ A1 ∪{∞} ((1, 0) getting mapped to ∞ ofc)
Our "fractional linear transformation" is given by
| ϕ : A1 ∪{∞} | → A1 ∪{∞} | (1) | ||
| x |
 (ax + b)∕(cx + d) (ax + b)∕(cx + d) | (2) | ||
| ad - bc | ≠0 | (3) |
A few things to note here.
FIRSTLY: Implicit here is that we send -d∕c to ∞. Singularity=infinity. Ez.
SECONDLY, for complex analysis fans, if k = ℂ, these are Mobius
transformations! .... idk what else to say about that. HELP ME, starsofcurtains!
FUG.
THIRDLY, I'm kinda wondering why we had to make the "affine identification" in order to define the map. I mean, couldn't we just have said this?
| ϕ : P1 | → P1 | (4) | ||
| (x,y) |
 (ax + by)∕(cx + dy) (ax + by)∕(cx + dy) | (5) | ||
| i.e.(x,y) |
 (ax + by,cx + dy) (ax + by,cx + dy) | (6) | ||
| (7) |
Well, admittedly, that does make some parts of the exercise harder (e.g.
finding an inverse). So the affine indentification is maybe not necessary but
helpful. OK!
FOURTHLY, I'm going to assume that c≠0 (which means thanks to ad-bc≠0,
we also have d≠0. Obviously the case c = 0 actually makes things easier, so no
need to worry about that.
OKAY: So the goal of part A is to show that ϕ is a morphism. Been
a while since we've done one of these morphism checks. Let's start by
finding an inverse set map. To do that, we'll jump back to good old high
school PreCalc. How do we do this? Set it equal to y and solve? OK.
| y | = (ax + b)∕(cx + d) | ||
 y(cx + d) y(cx + d) | = ax + b | ||
 ycx + yd ycx + yd | = ax + b | ||
 ycx - ax ycx - ax | = b - yd | ||
 x(yc - a) x(yc - a) | = b - yd | ||
 x(yc - a) x(yc - a) | = b - yd | ||
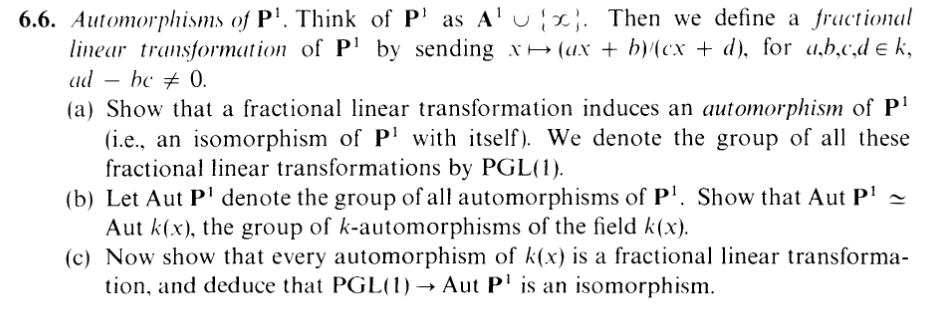
 x x | = (-dy + b)∕(cy - a) | ||
Holy jesus mother of god. In my notes I fucked this up so badly that I got
x = (cy -a)∕(dy -b). WHAT THE FUCKING FUCK LOL. I was sleepy, ok?
In any case, the above gives us an inverse for ϕ... and it happens to be another
"fractional linear transformation"! Let's call this map δ:
| δ : A1 ∪{∞} | → A1 ∪{∞} | ||
| y |
 (-dy + b)∕(cy - a) (-dy + b)∕(cy - a) |
This map sends a∕c to ∞. And, btw, you may be wondering "where do either
of these maps send ∞?" Well, in order to make these inverses of each other, we're
forced to set
| ϕ(∞) | = a∕c | ||
| δ(∞) | = -d∕c | ||
And those choices are pretty sensible if you look at the original definitions and
think of it from a limits standpoint. You guys see it? HELP ME, burypink!
FUCK.
Well, anyway, IT WORKS. ϕ is a bijection. NOW: All I have to do is show that ϕ is continuous and... morphic...?, and I'm done. "Ummm what about δ?" Psshh, δ is a fractional linear transformation just like ϕ, so the same arguments that apply to ϕ will apply to delta.
OK: I sort of lied. I'm actually going to show that ϕ is a closed map (which translates to δ is continuous), but that's equivalent in the end. Okay: Let's say that f ∈ k[x,y]. I'd like to make a polynomial f′ so that the image of ϕ satisfies f′ exactly when the preimage satisfies f. This is sufficient to show that ϕ is closed (ϕ(Z(f1,…,ft) = Z(f1′,…,f2′)). Well, I LIED AGAIN, but let's go forth with this as our motivation.
Let's let (X, 1) ∈ P1. Hence, from the A1 ∪{∞} perspective, we're assuming that X≠∞. Let Y = ϕ(X). Thanks to X≠∞, we can assume that Y ≠a∕c. OK. Let's set
| g(x) | = f(x, 1) |
So ofc
| f(X, 1) | = 0 | ||
| ⇔g(X) | = 0 |
Now, letting d = deg g I'm gonna define
| f′(y) | = g(
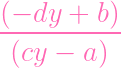 )(cy - a)d )(cy - a)d |
So, f′ is an element in k[y]. And note that
| f′(Y ) | = 0 | |||||
⇔g(
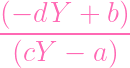 ) = 0 ) = 0 | or (cY - a)d = 0 | |||||
⇔g(
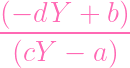 ) ) | = 0 | (since Y ≠a∕c) | ||||
| ⇔g(X) | = 0 |
yaaay, we can homogenize the polynomial f′ and thus we have a "counterpart"
for f.... For all the points that are NOT ∞. What about infinity THOUGH.
What if (1, 0) = ∞ satisfies f? Would its image (a∕c) satisfy our homogenized
f′? I almost went on a struggle to figure this out, until I realized: WHO CARES.
Singletons are closed in P1, so we can just union in a∕c and the resulting set still
remains closed. CLOSED SET MAP TO CLOSED SETS. ϕ IS CLOSED (δ is
cont). WEEEEEEEE. (By the same argument δ is closed so ϕ is cont).
So now I just have to show that our ϕ is a morphism. It's been a while, so here's
the definition of morphism:

As for regular functions, here's the definition for quasi-projective varieties:
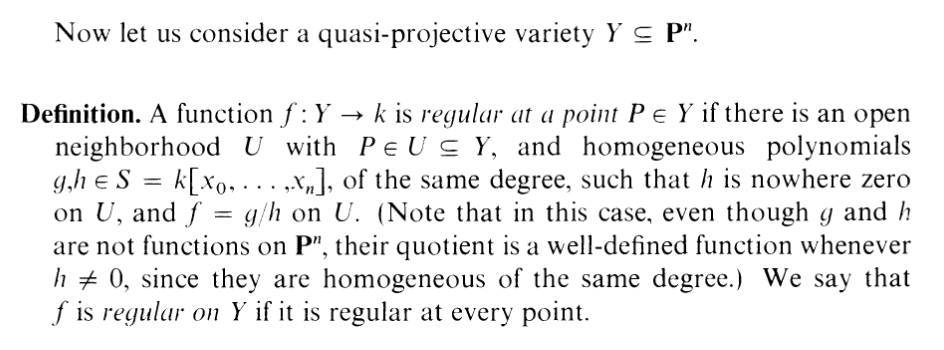
well, this is super tricky if you try and use the original definition (2) (WTF do
you do with ∞ and the singularity). HOWEVER, I think this is where my
alternate definition (6) comes in and, it's pretty easy to see that it trivially works
(I won't even go through the proof). DUN.
B
 errrrr..... Look at Corollary 6.12:
errrrr..... Look at Corollary 6.12:

yea.... It's just (i) ⇔ (iii), rite?. THERE'S NO WORK TO DO HERE LOL.