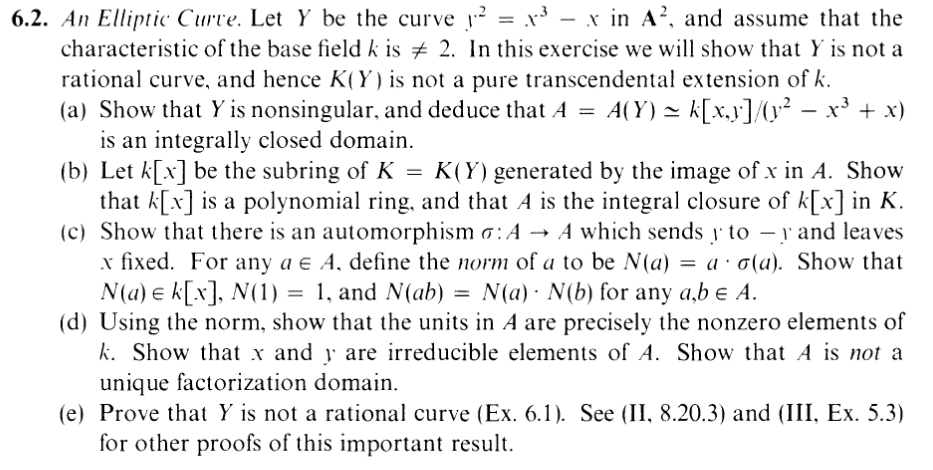
A

Have you ever wondered about elliptic curves? Elliptic curves show up in all sorts of applications, such as cryptography, and form the basis of many algebraic geometry problems. In particular, Section I.6.2 from Algebraic Geometry, by Robin Hartshorne explores this fascinating topic. It starts by considering whether a certain elliptic curve, given by y² = x³ -x. The previous section of that book, section five, connects the concept of the Jacobian matrix with singularity. Therefore, if we take the Jacobian matrix, we obtain . Hence, a singular point would have to satisfy -3x² + 1 = 0 as well as 2y = 0. Furthermore, in the case that chark = 3, then this is impossible. This is because this gives 1 = 0 on the first equation. On the other hand, if we assume chark≠2, 3 we obtain x² = 1∕3 and y = 0. Nevertheless, this point has to be on the original curve. The original curve equation is 0 = y² - x³ + x. Therefore, if we plug in the identities, this reveals that 0 = 0 - (1∕3)x + x. Furthermore, 0 = (2∕3)x. Additionally, don't forget the assumption that chark≠2. This reveals that x = 0. However, this a contradiction of the statement that -3x² + 1 = 0. Therefore, there are no singular points. Consequently, Y is nonsingular. Next, we have to conclude that A is integrally closed. One of the big lessons in section 6 of Hartshorne's work is that nonsingular points on curves have integrally closed local rings. According to Theorem I.3.2 (Hartshorne 17), A_𝔪P is integrally closed when P is nonsingular. Furthermore, since every point is nonsingular, the localization is closed at every maximal ideal. However, according to Wikipedia ("Integrally closed domain"), this is equivalent to the fact that A itself is integrally closed. This fact begs the question: Is Y a rational curve? You might be wondering this. However, due to the fact that A is the integral closure of k[x] in K, the properties of the norm, and the fact that A is not a unique factorization domain, Y is not a rational curve.

B

The first reason that Y is not a rational curve is that A is the integral closure of k[x] in K. In section six, during a proof of Lemma 6.5, Hartshorne states, "We consider the subring k[y] of K generated by y. Since k is algebraically closed, y is transcendental over k, hence k[y] is a polynomial ring" (41). This symbolizes the fact that k[x] is polynomial ring. This is because we can apply the same logic in our situation. Following this, we have to show that A is the integral closure of k[x] in K. In other words, if I is the integral closure of k[x], then we want to show that I = A. First, the inclusion I ⊂ A is obvious. Furthermore, according to a solution online, "Since y² ∈ k[x],y ∈ k[x]. So A ⊂ k[x]" (Cutrone 24) Therefore, the reverse inclusion has been proven and this section is done.

C

The next reason that Y is not rational is due to the properties of the norm. The exercise first asks us to "show that there is an automorphism σ : A → A which sends y to -y and leaves x fixed" (Hartshorne 46). However, this is immediately obvious so there's nothing to do. On the other hand, the properties of the norm are more nontrivial to show. Let us start by showing N(a) ∈ k[x]. In other words, we want to get rid of the y terms, using the identity y² = x³ - x. Let's say that a = ∑ _iγ_ix^b_iy^c_i. Therefore, N(a) = a ⋅ σ(a). Furthermore, N(a) = [∑ _iγ_ix^b_iy^c_i][∑ _iγ_jx^b_j(-y)^c_j], where i,j go over the same index set. Consequently, we can write N(a) as made up of terms in the form. L₁ = γ_ix^b_iy^c_iγ_jx^b_j(-y)^c_j. This reveals that L₂ = (-1)^c_jγ _iγ_jx^b_i+b_jy^c_i+c_j. In the case that c _i + c_j is even, this represents the fact that ∃d : c_i + c_j = 2d. Therefore y^c_i+c_j = (y²)^d. Furthermore, this is equal to (x³ - x)^d. This gets rid of the y. So the even terms are safe. However, one may argue that c_i + c_j could be odd. To refute this point, let us assume without loss of generality that c_i is odd and c_j is even. In this situation, L₁ turns into L₁ = γ_iγ_jx^b_i+b_jy^c_i+c_j. On the other hand, since i,j range over the same index set, there's another term "opposite" to L₁, with i,j swapped. This term has the form L₂ = γ_jx^b_jy^c_jγ _ix^b_i(-y)^c_i. Therefore, L₂ = (-1)^c_iγ_iγ_jx^b_i+b_jy^c_i+c_j. Furthermore, L ₂ = -γ_iγ_jx^b_i+b_jy^c_i+c_j. This follows from the fact that c_i is odd. Thus, L₁ and L₂ cancel each other out. In total, we are left with a polynomial over x. This is equivalent to the statement that N(a) ∈ k[x]. The other two properties of N are obvious.

D

Finally, Y is not a rational curve is due to the fact that A is not a unique factorization domain. This can be foreshadowed using a fact that I will call "Lemma One." The statement of this made-up Lemma reads, N(a) ∈ k a ∈ k. The proof of this Lemma is left to the reader. Now we get to the main point. If a comes from A, we would like to show that it is a unit if and only if it is a nonzero element of k. Lemma One reveals one side of this implication. It reveals the "if" side of the implication. Therefore, we need to show the "only if" side. Hence, let us suppose that a ∈ A is a unit. This happens if and only if there is an element b that comes from A such that ab = 1. This implies that N(ab) = 1. Therefore, N(a)N(b) = 1. Furthermore, N(a) ∈ k and N(a) ∈ k. Thus, a ∈ k, thanks to Lemma One. Next, we have to show that x and y are irreducible elements of A. First, let us suppose that x = ab. In that case, if we apply N to both sides, this reveals that N(x) = N(ab). Therefore, N(x) = N(a)N(b). Furthermore, since N(x) = x by definition, this reveals that x = N(a)N(b). Moreover, since k[x] is a polynomial ring, we are allowed to assume that either N(a) or N(b) is a unit. Finally, applying Lemma One completes the proof. Likewise, there is an analogous argument for y. Next, we have to show that A is not a unique factorization domain. This follows from the fact that we can write y² as y ⋅ y. However, we can also write it as x³ - x, which factors as x(x + 1)(x - 1). One of these factorizations has an irreducible factor of y. In contrast, the other has an irreducible factor of x. Hence, these are distinct factorizations of y². Consequently, A is not a unique factorization domain.

E

"Umm, Mr. DickBallAssBitchFaggot? Why is this sample essay so boring?" Ah, that's a good question, Jenny. That's funny, this paper got an A, and came from one of my best students. What do you think, class? You all think it's boring? ...Interesting. Okay. How about we try an exercise? Everyone put your head down and close your eyes.... Everyone ready? Okay, now, think of the ocean, the waves, the bubbles–Aiden, I don't see your head down..... Thank you–the ocean, the waves, the bubbles, the great big sky... Breathe in, breathe out... clear your mi–Aiden, Tessa, I'll wait.... Thank you. Now breathe in... breathe out... Yes, the great big sky... All you see is the great big sky. And what do you see up there? A holy figure is coming down from the sky, descending onto us base beings. What does it look like? Is it a bird? A plane? No, no, it's something greater. You start hearing that ominous chant, Dies irae, dies illa, Solvet saeclum in favilla, as the great figure gets closer and closer. And thus, out of the clouds, the figure begins revealing itself. First, you see an intro. Then, swooping down one-by-one, 1..2..3 body paragraphs. And finally, that unmistakeable, wonderous tail: The conclusion. Yes, indeed, it's the 5-paragraph essay! The greatest format ever invented by man. I call it "The God Format". Reading 5-paragraph essays has been a widely beloved joy for intelligent readers throughout history, since the birth of Christ. Heh, you know, back when I was your age, we used to have 5-paragraph potlucks. Everyone would come together and bring their own 5-paragraph essay, and we'd read all of them, and peer-review them, and ohohoho, it was so much fun! We'd argue through the night about which one of us had the best hook. You see? You might want to think twice before calling one of the ancient tenets of high school English classes all across America "boring". Well, I get your gripe, Jenny. Why all the rules, right? Intro with a hook, topic statement, thesis statement where you list your arguments, 3 body paragraphs each with 3 supporting pieces of evidence, followed by a conclusion, and on top of that, making sure your essay follows MLA format, satisfies my page requirement, and worrying about spelling, as well as grammar specificities such as not starting sentences with conjunctions, avoiding sentence fragments, run-on sentences, making sure your commas and semicolons are used properly, and avoiding casual language. When I was in high school, I used to feel like it made my writing stiff, just like you guys. But y'know what? As I grew up, I started to appreciate the format more. Here's the thing: You guys are young, creative, and all that, and we want to shape you into good writers. But you have to learn the rules before you can break them. Yes, advanced writers break these rules all the time. But you all aren't writers yet. You're all amateurs, and that's okay, but you first have to understand how to organize arguments, and fit them into a format presentable in a formal setting. Indeed, at this tender age when your wild writing voice is being developed, the thing we want to make sure we pin down is... formality! ...You all seem unconvinced. Well, you'll have to excuse me, I'm getting old. You see, well, I like to chastise you teenagers for being on your devices all the time, but I don't realize that in that horrific, festering pot that is the internet, you young folks are actually writing all the time. Arguments in Youtube comment sections, cringy tirades on your Twitlongers and blogs, egotistical late night rants to your Discord buttbuddies, nervous personal website bios, sermons in MMO town squares—all that unhinged transfer of your innards into text, spread out in dark, personal corners of the web—some of the things you've written in there are probably beautiful, wild, radical. Indeed, there is a well of fortune in that miserable, narcissistic anthology of yours. You have this unbelievable capability to produce awful yet passionate text on the fly, to a level that even established novelists would be jealous of. Maybe you are writers. Maybe I shouldn't let that go to waste. Maybe my job should be to try and tap into that energy, bring it out in class, and mould it into something more presentable. But, you see, in those things you type out, you touch too many icky, edgy topics. You use all these newfangled colloquialisms that would never be accepted in an academic setting. And it's all formatted and structured in such an unpredictable way that I can't engage with it. That streak of creativity you express in your personal life shall have no relation to what we do in this class. This class is SAFE. We want to keep everything clean. We make you wear masks and sanitize your desks, after all. Can you imagine cussing in an argumentative essay? That's no way to argue a point. Actually, you'll get expelled if you do that, hahahaha. You know what, Jenny, I'll admit it. Teenagers probably have far more creativity than old shitters like me. If I let you all write with no restrictions, you'd wipe the floor with anything I could produce. No, you'll never see a sample of my own writing in this class. It may be hypocritical to force you to write all this crap and not produce anything of my own along with you, but I'm sorry, I don't know how to write. You kids would probably lose all faith in my teaching abilities if you saw my godawful writing. Folks, I haven't written a single thing in the past 20 years. If I were actually a good writer myself, I might join you in the trenches and write essays alongside you all, instead of pointing to the same sample essays and prompts every year embedded in ancient powerpoints. But who do you think I am? Alexander, throwing away a helmet of water from Zephyrus? Nay, I'm not one of you. I'm above you. But I care about you. I want you all to go FAR. In conclusion, the fact that Y is not rational follows from exercise 6.1 (Hartshorne 46).

Works Cited

Cutrone, Joe, and Nick Marshburn. Algebraic Geometry By: Robin Hartshorne Solutions.

Hartshorne, Robin. Algebraic Geometry. Springer, 1977.

“Integrally closed domain" Wikipedia, Wikimedia Foundation, 10 July 2021, https://en.wikipedia.org/w/index.php?title=Integrally_closed_domain&oldid=1032998236